Chapter 8: Option pricing

Chapter learning Objectives

Upon completion of this chapter you will be able to:

• explain the principles of option pricing theory
• determine, using published data, the five principal drivers of option value (value of the underlying, exercise price, time to expiry, volatility and the risk-free rate)
• discuss the underlying assumptions, structure, application and limitations of the Black-Scholes model
• recognise real options embedded within a project, and classify them as one of the real option archetypes
• use the principles of the Black-Scholes model to explain the value of real options to delay, expand, redeploy and withdraw in investment projects and calculate the value of the options.
• use option pricing theory to calculate credit spreads.

1 The principles of option pricing theory

Option terminology

Option value

The key aspect to an option’s value is that the buyer has achoice whether or not to use it. Thus the option can be used to avoiddownside risk exposure without foregoing upside exposure.

The value of an option is made up of two components. These are illustrated below for a call option:

The intrinsic value

The intrinsic value looks at the exercise price compared with the price of the underlying asset.

• The value of the call option will increase as the share price increases. Conversely a lower exercise price would also give a higher option value.
• An option can never have a negative intrinsic value. If the option is out of the money, then the intrinsic value is zero.
• On the expiry date, the value of an option is equal to its intrinsic value.

The time value

• Time to expiry.
  • As the period to expiry increases, the chance of a profit before the expiry date grows, increasing the option value.
• Volatility of the share price.
  • The holder of a call option does not suffer if the share price falls below the exercise price, i.e. there is a limit to the downside.
  • However the option holder gains if the share price increases above the exercise price, i.e. there is no limit to the upside.
  • Thus the greater the volatility the better, as this increases the probability of a valuable increase in share price.
• Risk-free interest rate.
  • As stated above, the exercise price has to be paid in the future, therefore the higher the interest rates the lower the present value of the exercise price. This reduces the cost of exercising and thus adds value to the current call option value.
  • Alternatively, since having a call option means that the share purchase can be deferred, owning a call option becomes more valuable when interest rates are high, since the money left in the bank will be generating a higher return.

Summary of the determinants of call option prices:

Test your understanding 1

Complete the following table for put options.

Summary of the determinants of call option prices:

Test your understanding 2

A pension fund manager is concerned that the value ofthe stock market will fall. Suggest an option strategy he could use toprotect the fund value.

The drivers of option value in practice

Introduction

As discussed above, the main drivers of option value are as follows:

• value of the underlying asset
• exercise price
• time to expiry
• volatility
• risk-free rate.

Determining these figures in practice is discussed below.

Value of the underlying asset

• For quoted underlying assets a value can be looked up on the market. Most markets give prices for buying and selling the underlying asset. A mid-price is usually used for option pricing.

  For example, if a price is quoted as 243–244 cents, then a mid-price of 243.5 cents should be used.

• In the case of unquoted underlying assets a separate exercise must be undertaken to value them.

  For example, suppose an unquoted company has issued share options to employees as part of their remuneration package. To value these call options (e.g. for disclosure or taxation purposes) one must first value the shares using, e.g. P/E ratios.

Exercise price and time to expiry

Both the exercise price and expiry date are stated in the terms of the option contract.

Volatility

• Volatility represents the standard deviation of day-to-day price changes in a security, expressed as an annualised percentage. Two measures of volatility are commonly used in options trading: historical and implied.
• Historical volatility can be measured by observing price changes of a security over a period of time. It is not necessarily a forecast of future volatility, but can be used to determine the option price.
• Implied volatility can be calculated by taking current quoted options prices and working backwards.

A common approach to calculating historical volatility is as follows:

(1) Calculate the daily return using (current price/previous day’s price) or P_n/P_n–1.

(2) Take the log of each ‘return’ to convert into a continuous return.

(3) Calculate the standard deviation of the logs to get a daily volatility.

(4) Annualise the result.

Volatility calculation

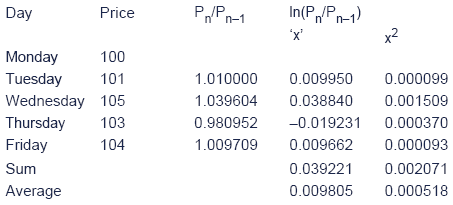
In order to calculate the volatility (standard deviation) we need to use these two final figures.

Volatility = square root of (the average value of x² less the square of the average value of x)

= √ (0.000518 – 0.009805²)

= 0.0205 or approximately 2%

Assuming 260 trading days on the market, Annualised volatility = daily volatility × √260 = 0.33 or 33%.

The method for calculating the continuous return in the aboveexample may be unfamiliar to you. The basic idea is that instead ofdividing a time period into years or weeks or days for discountingpurposes we can discount continuously. To get the same answer eitherway, we need to set continuous rate = ln (1 + discrete rate)

For example, if the discrete rate is 10% p.a., then a continuous rate is given by

Continuous rate = ln1.10 = 0.0953 or 9.53%.

Discount factors using continuous rates are given by DF = e^-it where i is the continuous rate and t the time period.

Risk-free rate

• The risk-free rate is the minimum return required by investors from a risk-free investment.
• Treasury bills or other short-term (usually three months). Government borrowings are regarded as the safest possible investment and their rate of return is often given in a question to be used as a figure for the risk-free rate.

2 The Black-Scholes option pricing model

Introduction

The Black-Scholes model values call options before the expiry date and takes account of all five factors that determine the value of an option.

Using the Black-Scholes model to value call options

Value of a call option = P_a N(d₁) – P_eN(d₂)e^–rt

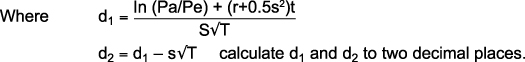
Note: The formula is daunting, but fortunately you do notneed to learn it, as it will be given in the examination paper. You needto be aware only of the variables which it includes, to be able to plugin the numbers.

The key:

P_a = current price of underlying asset (e.g. share price)

P_e = exercise price

r = risk-free rate of interest

t = time until expiry of option in years

s = volatility of the share price (as measured by the standard deviation expressed as a decimal - see explanation below)

N(d) = equals the area under the normal curve up to d (see normal distribution tables)

e = 2.71828, the exponential constant

In = the natural log (log to be base e)

P_ee^-rt = present value of the exercise price calculated by using the continuous discounting factors.

Measures of volatility - standard deviation and variance

The measure of volatility used in the Black-Scholes model is the annual standard deviation (s), expressed as a decimal.

Exam questions may quote volatility in terms of the "variance",which is the square of the standard deviation. In this case, take thesquare root of the given variance figure to give the volatility in thecorrect terms for the Black-Scholes formula.

Alternatively, monthly, or weekly, standard deviations may bequoted. To convert from a monthly standard deviation to an annualfigure,

• square the monthly standard deviation
• multiply by 12
• take the square root of the result.

This will now be the annual standard deviation figure as required.

Normal distribution tables

Recap of normal distributions

Extract from standard normal distribution table

This table can be used to calculate N(d₁), the cumulative normal distribution function needed for the Black-Scholes model of option pricing.

• If d₁ > 0, add 0.5 to the relevant number above.
• If d₁ < 0,="" subtract="" the="" relevant="" number="" above="" from="">

For example if d₁ is 1.05, N(d₁) = 0.3531 + 0.5 = 0.8531.

Note

N(d) = Is the area under the normal curve up to d in the shaped area of the figure below.

Illustration of the Black-Scholes model

Calculate the value of the above call option.

Solution

Step 1: Calculate d₁ and d₂.

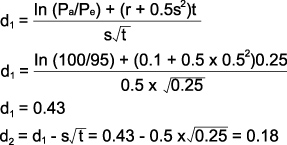
Step 2: Use normal distribution tables to find the value of N(d₁) and N(d₂).

N(d₁) = 0.5 + 0.1664 = 0.6664

N(d₂) = 0.5 + 0.0714 = 0.5714

Step 3: Plug these numbers into the Black-Scholes formula.

Value of a call option = P_a N(d₁) – P_eN(d₂)e^-rt

Note

This can be split between the intrinsic value of $5 (100 – 95) and the time value which is $8.70.

Test your understanding 3

Suppose that the risk-free rate is 5% and the standard deviation ofthe return on the share in the past has been estimated as 34.64%.

Estimate the value of a six-month call option at an exercise price of $1.48 (current share price = $1.64).

Using the Black-Scholes model to value put options

If you have calculated the value of a call option usingBlack-Scholes, then the value of a corresponding put option can be foundusing the put call parity formula.

The put call parity equation is on the examination formula sheet:

Put call parity P= c - P_a + P_e× e^–rt

Step 1: Value the corresponding call option using the Black-Scholes model.

Step 2: Then calculate the value the put option using the put call parity equation.

Black-Scholes model: value put options

Returning to the earlier example of B plc, where the current shareprice is $100, exercise price is $95, the risk-free rate of interest is10%, the standard deviation of shares return is 50% and the time toexpiry is three months, calculate the value of a put option.

Solution

• Step 1: We have already calculated the value of the call option at $13.70.
• Step 2: Using put call parity equation:

Put call parity P= c - P_a + P_e×e^–rt

Value of a put = 13.70 - 100.00 + 92.65

= $6.35

Test your understanding 4

Using the information given in TYU 3, calculate the value of the corresponding put option.

Underlying assumptions and limitations

The model assumes that:

• The options are European calls.
• There are no transaction costs or taxes.
• The investor can borrow at the risk-free rate.
• The risk-free rate of interest and the share’s volatility is constant over the life of the option.
• The future share-price volatility can be estimated by observing past share price volatility.
• The share price follows a random walk and that the possible share prices are based on a normal distribution.
• No dividends are payable before the option expiry date.

In practice these unrealistic assumptions can be relaxed and thebasic model can be developed to reflect a more complex situation.

Application to American call options

One of the limitations of the Black-Scholes formula is thatit assumes that the shares will not pay dividends before the optionexpires. If this holds true then the model can also be used to valueAmerican call options.

In fact, if no dividends are payable before the option expiry date,the American call option will be worth the same as a European calloption.

You will not be asked to value American call options on shares that do pay dividends or American put options using the Black-Scholes model.

Application of Black-Scholes model

Going back to our exercise about B plc, where we calculated thatthe value of the call option was $13.70 with three months to expiry andassuming that this is an American option and the holder wants to closethe option today he has two basic choices:

(1) exercise today and benefit by the intrinsic value of $5.00 or

(2) sell the option back to the market and receive $13.70 (intrinsic value plus time value).

Therefore if the American option-holder exercises early he willforfeit the time value. Quite simply the option is ‘worth more alivethan dead’. The right to exercise an American call early is irrelevantand the option will be exercised on the expiry date just like aEuropean option.

Therefore the values of European and American call options on shares not paying dividends are equal.

You will not be asked to value American call options on shares that do pay dividends or American put options using the Black-Scholes model.

Application to shares where dividends are payable before the expiry date

The Black-Scholes formula can be adapted to call optionswith dividends being paid before expiry by calculating a ‘dividendadjusted share price’:

• Simply deduct the present value of dividends to be paid (before the expiry of the option) from the current share price.
• P_a, becomes P_a – PV (dividends) in the Black-Scholes formula.

Illustration 1: The Black-Scholes option pricing model

You have been asked to value call options in D plc.

• The options are due to expire in five months’ time. However a dividend of 40 cents is due to be paid in three months’ time.
• The current share price is $10.
• The risk-free rate is 10%.

What value should be used for the dividend-adjusted share price?

Solution

Three months’ time t = (3/12) = 0.25 of a year, r = 0.10.

• The present value of the dividends is equal to:

  40e^–rt = 40e ^{– (0.1 × 0.25)} = 39.01 say 39 cents.

• So P_a – PV (dividends) = $10.00 – 0.39 = $9.61, is used in the Black-Scholes model.

Test your understanding 5

A call option on V plc’s shares has the following details:

• Exercise price $50
• Current share price $40
• Risk-free rate 8%
• Time to expiry 3 months
• Dividend of $2 per share to be paid in three months’ time.

Calculate a dividend-adjusted share price for use in the Black-Scholes option pricing model.

Introduction to the Grabbe Variant

Application to foreign currency options – the ‘Grabbe variant’

• The risk-free interest rate is a necessary component of the Black-Scholes model to incorporate the time value of money.
• A complication that arises with currency options is that there are now two interest rates – one for each currency. These can be incorporated by using the predicted forward rate in the "Grabbe variant" formula.
• You are not expected to learn the Grabbe variant formula for the exam, and it is not given on the formula sheet. The formula and illustration presented below are purely for illustration.

Value of a currency call option = e ^-rT [F N(d₁) - X N(d₂)]

Value of a currency put option = e ^-rT [X N(-d₂) - F N(-d₁)]

Where d₁ = (ln (F/X) + ½s²T)/s√T

d₂ = d₁ -s√T.

F = forward rate, calculated using interest rate parity (see below)

X = exercise rate

r = domestic interest rate (as usual)

F and X need to be quoted as the price of the foreign currency(i.e. ‘direct’ quotes) but indirect rates are used in the interestrate parity formula:

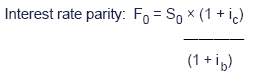
Illustration of the Grabbe Variant formula

A US firm is suing a French rival over patent infringement and willfind out in 9 months' time whether it has been successful, in whichcase an immediate receipt in euros will crystallise. The treasurer hasdecided to hedge using currency options.

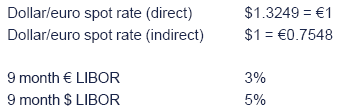
Annual volatility of the Euro against the dollar 25%.

Required:

Calculate the value of a 9 month Euro/dollar putoption with an exercise price of $1.3249 = €1 (i.e. currently “atthe money”).

Solution

Step 1: Calculate the forward rate using interest rate parity (usually used with indirect exchange rates).

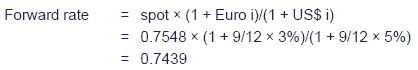
Quoted as a direct rate this is 1/0.7439 = 1.3443

Step 2: Calculate d₁ and d₂ using direct exchange rates.

Step 3: Use normal distribution tables to find the value of N(-d₁) and N(-d₂).

N(-d₁) = 0.5 - 0.0695 = 0.4305

N(-d₂) = 0.5 + 0.0164 = 0.5164

Step 4: Plug these numbers into the Black-Scholes formula.

Value of a put option = e ^-rt [X N(-d₂) - F N(-d₁)]

= e ^{- (0.05 × 0.75)} [1.3249 × 0.5164 – 1.3443 × 0.4305]

= 0.96319 × 0.105457

= $0.10157 or 10.157 cents per euro.

Delta and delta hedges

The figure N(d₁) is known as delta. Delta measures thechange in option value which would result from a $1 change in the valueof the underlying asset (e.g. share).

An investor can eliminate the risk of his shareholding by constructing a ‘delta hedge’.

• An investor who holds a number of shares and sells (an option writer) a number of call options in the proportion dictated by the delta/the hedge ratio ensures a hedged portfolio. N.B. A hedged portfolio is one where the gains and losses cancel out against each other.
• Number of option calls to sell = Number of shares held/N(d₁).
• Alternatively, if you have already written call options, then a delta hedge can be constructed by buying shares.
• Number of shares to hold = Number of call options sold × N(d₁).

Because share prices change continuously in the real world, thevalue of delta also changes continuously. Therefore, the investor whowants to maintain a risk neutral position will have to continuouslyadjust the balance of options and shares in his portfolio. This processis known as "dynamic delta hedging".

Example of a delta hedge

Assuming a call option currently has a delta of 0.5, let use it toconstruct a hedged portfolio for an investor who holds 100 shares.

The investor in shares will find out how many call options he will have to (write) sell.

So if you had 100 shares we would need to sell (100 shares/.5) =200 calls to construct a delta hedge. The number of options will exceedthe number of shares unless the delta is 1, then they would be an equalnumber of each.

The call option writers (the seller of the call options) will find out how many shares they will have to buy.

If the share price increases by 10 cents, the call options increaseby 5 cents. However as we have sold the call option our portfoliodecreases by 5 cents for every call option sold.

However, the difficulty is that the delta value is not at a constant. It changes as the share price changes.

Suppose that the 10 cents move in the share price caused the deltato move to 0.7. The option writer will need to buy 200 calls × .7 = 140shares in order to hedge the position, i.e. 40 extra shares.

The portfolio will need rebalancing as the delta value changes. Thefrequency of this depends on the rate of change of delta, measured bygamma.

Gamma, vega, rho and theta

Delta measures the sensitivity of the option value to changes in the value of the underlying asset (explained in detail above).

Sensitivities to other factors in the Black Scholes formula are denoted by other Greek letters as follows:

Gamma - measures the rate of change of delta as the underlying asset's price changes.

Vega - measures the change in option value caused by a 1% change in the volatility.

Rho - measures the sensitivity of the option value to changes in the risk free rate of interest.

Theta - measures the rate of decline in the value of the option caused by the passage of time.

3 Identifying real options in investment appraisal

Introduction

• Flexibility adds value to an investment.
• Financial options are an example where this flexibility can be valued.
• Real options theory attempts to classify and value flexibility in general by taking the ideas of financial options pricing and developing them.
• Conventional investment-appraisal techniques typically undervalue flexibility within projects with high uncertainty.

More detail on valuing flexibility

• Flexibilty adds value to an investment:
  • For example, if an investment can be staggered, then future costs can be avoided if the market turns out to be less attractive than originally expected.
  • The core to this value lies in reducing downside risk exposure but keeping upside potential open – i.e. in making probability distributions asymmetric.
• Financial options are an example where this flexibility can be valued.
  • A call option on a share allows an investor to ‘wait and see’ what happens to a share price before deciding whether to exercise the option and will thus benefit from favourable price movements without being affected by adverse movements.
• Real options theory attempts to classify and value flexibility in general by taking the ideas of financial options pricing and developing them:
  • A financial option gives the owner the right, but not the obligation, to buy or sell a security at a given price. Analogously, companies that make strategic investments have the right, but not the obligation, to exploit these opportunities in the future.
  • As with financial options most real options involve spending more up front (analogous to the option premium) to give additional flexibility later.
• Conventional investment-appraisal techniques typically undervalue flexibility within projects with high uncertainty.
  • High uncertainty within a NPV context will result in a higher discount rate and a lower NPV. However, with such uncertainty any embedded real options will become more valuable.

Different types of real option

There are many different classifications of real options. Most can be summarised under the following generic headings:

Options to delay/defer

The key here is to be able to delay investment without losing the opportunity, creating a call option on the future investment.

Illustration 2: Identifying real options in investment appraisal

For example, establishing a drugs patent allows the owner of thepatent to wait and see how market conditions develop before producingthe drug, without the potential downside of competitors entering themarket.

(Note: drugs patents was the subject of a past examinationquestion on this area. However, there is some debate whether or notpatents are real options. This debate is outside the scope of thesyllabus.)

Options to switch/redeploy

It may be possible to switch the use of assets should market conditions change.

Illustration 3: Identifying real options in investment appraisal

For example, traditional production lines were set up to make oneproduct. Modern flexible manufacturing systems (FMS) allow the productoutput to be changed to match customer requirements.

Similarly a new plant could be designed with resale and/or other usesin mind, using more general-purpose assets than dedicated to alloweasier switching.

Illustration 4: Identifying real options in investment appraisal

For example, when designing a plant management can choose whetherto have higher or lower operating gearing. By having mainly variablecosts, it is financially more beneficial if the plant does not have tooperate every month.

Options to expand/contract

It may be possible to adjust the scale of an investment depending on the market conditions.

Options to abandon

If a project has clearly identifiable stages such that investmentcan be staggered, then management have to decide whether to abandon orcontinue at the end of each stage.

Illustration 5: Identifying real options in investment appraisal

When looking to develop their stadiums, many football clubs face the decision whether to build a one- or a two-tier stand:

• A one-tier stand would be cheaper but would be inadequate if the club’s attendance improved greatly.
• A two-tier stand would allow for much greater fan numbers but would be more expensive and would be seen as a waste of money should attendance not improve greatly.

Some clubs (e.g. West Bromwich Albion in the UK) have solved thisproblem by building a one-tier stand with stronger foundations anddesigned in such a way (e.g. positioning of exits, corporate boxes,etc.) that it would be relatively straightforward to add a second tierat a later stage without knocking down the first tier.

Such a stand is more expensive than a conventional one-tier standbut the premium paid makes it easier to expand at a later date when(if!) attendance grows.

Illustration 6: Identifying real options in investment appraisal

Amazon.com undertook a substantial investment to develop itscustomer base, brand name and information infrastructure for its corebook business. This in effect created a portfolio of real options toextend its operations into a variety of new businesses such as CDs,DVDs, etc.

Test your understanding 6

Comment on a strategy of vertical integration in the context of real options.

Test your understanding 7

A film studio has three new releases planned for the Christmas periodbut does not know which will be the biggest hit for allocatingmarketing resources. It thus decides to do a trial screening of eachfilm in selected cinemas and allocates the marketing budget on the basisof the results.

Comment on this plan using real option theory.

4 Valuing real options

Introduction

Valuing real options is a complex process and currently a matter ofsome debate as to the most suitable methodology. Within the P4 syllabusyou are expected to be able to apply the Black-Scholes model to realoptions.

Using the Black-Scholes model to value real options

The Black-Scholes equation is well suited for simple realoptions, those with a single source of uncertainty and a single decisiondate. To use the model we need to identify the five key input variablesas follows:

Exercise price

For most real options (e.g. option to expand, option to delay), thecapital investment required can be substituted for the exercise price.These options are examples of call options.

For an option to abandon, use the salvage value on abandonment. This is an example of a put option.

Share price

The value of the underlying asset is usually taken to be the PV ofthe future cash flows from the project (i.e. excluding any initialinvestment).

This could be the value of the project being undertaken for a calloption (e.g. option to expand, option to delay), or the value of thecashflows being foregone for a put option (option to abandon).

Time to expiry

This is straightforward if the project involves a single investment.

Volatility

The volatility of the underlying asset (here the future operatingcash flows) can be measured using typical industry sector risk.

Risk-free rate

Many writers continue to use the risk-free rate for real options.However, some argue that a higher rate be used to reflect the extrarisks when replacing the share price with the PV of future cash flows.

Illustration 7: Valuing real options

A UK retailer is considering opening a new store in Germany with the following details:

These figures would suggest that the investment should be rejected.However, if the first store is opened then the firm would gain theoption to open a second store (an option to expand).

Suppose this would have the following details:

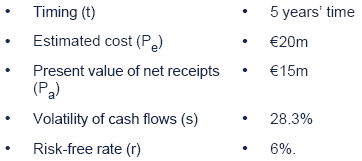
The value of the call option on the second store is then calculated as normal using Black-Scholes:

Step 1: Compute d₁ and d₂

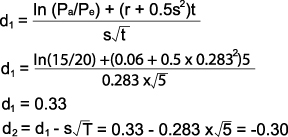
Step 2: Compute N(d₁) and N(d₂).

N(d₁) = 0.5 + 0.1293 = 0.6293

N(d₂) = 0.5 - 0.1179 = 0.3821

Step 3: Use formula.

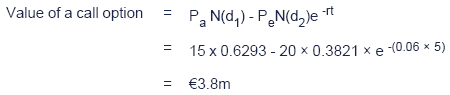
Summary

The project should thus be accepted.

Test your understanding 8

An online DVD and CD retailer is considering investing $2m onimproving its customer information and online ordering systems. This isjustified on the grounds that it will allow the business to extend therange of products offered. In particular the board are interested inselling gadgets and have estimated the following:

Advise the firm.

5 Using option pricing theory to calculate credit spreads

Explanation of credit spreads

For any bond the lender’s required/expected return will be made up of two elements:

• The risk free rate of return
• A premium (“the credit spread”) based on the expected probability of default and the expected loss given default - covered in the earlier chapter on WACC (when looking at cost of debt).

Option pricing theory (OPT) can be used to calculate these credit spreads and the risk of default.

How to use OPT to calculate credit spreads

Overview of the method

A key concept in this context is that shareholders can be viewed ashaving a call option on the company’s assets. By redeeming the debt,shareholders effectively acquire the assets of the company. Defaulthands the assets to the lenders.

Shareholders exercise this option if the value of the firm’sassets is at least equal to the redemption value. If not, then theoption is allowed to lapse, the company is handed over to the debtholders and the shareholders walk away.

Not defaulting thus corresponds to the call option being in the money at expiry.

(A corresponding view is that the lenders have sold a put optionunder which the company is sold to them for more than it's worth –they give up the full redemption value in exchange for the companyassets)

Numerical illustration

Suppose OPT Inc has the following capital structure

Furthermore, suppose that:

• The (discrete) risk-free rate r_f = 5%
• Volatility = s = 25%

Next, let us start by considering the case if the debt were risk free.

(1) The yield to the lender would be 5% and so the redemption value in 5 years should be 60 (1.05)⁵ = 76.577

(2) The equity can then be valued as a call option on the company’s assets:

• Value of underlying asset = 100
• Exercise price = redemption value of debt = 76.577
• Volatility = s = 25%
• Continuous risk free rate = ln(1.05) = 0.04879 = 4.879%
• t = 5

Using this with the Black Scholes formula gives a call value of 44

(3) The current value of debt can then be valued as the total asset value less the value of the equity:

Value of debt = 100 - 44 = 56

This is the wrong answer as we know the debt to be worth 60.The problem is that lenders will require a risk adjusted return higherthan 5% and hence a higher redemption value than 76.577

(4) Using the “solver” or “goal-seek” function in a spreadsheet thiscalculation can be reversed to determine what redemption value doesgive the current value of debt as 60. The required redemption value is84.

The required return of the lender is thus given by

1 + r = (84/60)^1/5 = 1.07

The lender thus requires 7%, giving a credit spread of 2% over the risk free rate.

Note the return and spread have been calculated using discrete returns. A continuous approach would be as follows:

• Required return of lender = (1/5) × ln(84/60) = 0.06729 or 6.729%
• Credit spread = 0.06729 - 0.04879 = 0.0185 or 1.85%.

The probability of default

It can be shown that the probability of a call option ending in the money can be calculated as N(d*), where d* is given by

Where µ = (continuous return) on the company’s assets

Suppose in our OPT Inc example that

• The (discrete) market return r_m = 9²/₃%
• Asset beta = β_a = 0.6

From this data we can use CAPM to calculate a required return on the company’s assets as

K_a = r_f + β_a(r_m - r_f) = 0.05 + 0.6(0.09666 – 0.05) = 0.078 or 7.8%

This can be converted into a continuous return as µ= ln(1.078) = 0.0751 or 7.51%

Substituting this into the above formula for d*:

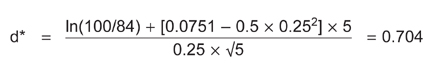
The probability of NOT defaulting is N(0.704) = 0.758

The probability of default is then 1 – 0.758 = 0.242 or 24.2%.

Calculation of credit spreads using probability theory

Alternatively, credit spreads can be calculated using probability theory.

Based on the lender's assessment of credit risk and probability ofdefault (covered in the above Expandable Text and in the earlier chapteron WACC), the lender sets the interest rate such that the expectedreturn from the debt is at the appropriate level for the level ofperceived risk.

Using prob. theory to value credit spreads

Panda plc is a UK sportswear company which has expanded rapidlyover the last 10 years. Its assets are now valued at £55m. In order tofund its most recent project, a new manufacturing facility in a deprivedarea of the West Midlands, the directors have decided to borrow £10mfrom the ITCD Bank plc. The money will be repayable in 1 year.

The interest rate quoted on the loan is 5.90% p.a., being base rateplus 65 basis points. The directors are unsure how this rate has beencomputed. The bank has provided them with the following information byway of explanation:

• Loan from ITCD Bank plc to Panda plc
• Loan value:   Â£10m     
• Asset value:   Â£55m
• Standard deviation of returns: 40% p.a.    
• Base rate:   5.25% p.a.
• Expected level of recovery on default: 70%
• Panda plc Credit Rating: AA      
• Credit spread on a 1 year loan: 65 basis points

Required:

Show how the interest rate on the loan of 5.90% has been derived.

Solution

With assets of £55m and a loan of £10m, the bank will havecalculated the likelihood of Panda plc’s assets falling below £10m inthe next year, as follows:

Standard deviation = 40%, which (on an average asset value of £55m) translates to a value of £22m.

Hence, the loan value of £10m lies £45m, or 45/22 = 2.045 Standard Deviations below the mean.

Using normal distribution tables, the area under the normal curvebetween the mean and this point is 0.4796. Thus (including theproportion of the curve lying above the mean) there is a 0.9796 chance(97.96%) of Panda plc’s asset values exceeding the £10m loan value in1 year. This means that there is a 0.0204 chance (2.04%) of default, inwhich case the bank predicts that only 70% of the loan will berecoverable.

The bank will now calculate an interest rate such that the expectedreceipt in 1 year exactly covers the amount borrowed, as follows:

Assuming a base rate of 5.25%:

Value of £10m at time 1 (divide by 1.0525) =   Â£9,501,188

Total expected receipt = Expected value if paid + Expected value on default

Expected value if paid = 0.9796 x £9,501,188 =   Â£9,307,363
Expected value if default = 0.7 x 0.0204 x £9,501,188 =  Â£135,677
Total expected receipt =     Â£9,443,040

Hence £10m loan = £9,443,040 x [1 + i] hence i = 0.05898 or 5.90%, which is base rate plus 65 basis points as quoted.

Student Accountant article

Bob Ryan's August 2008 Student Accountant magazine article on "How lenders set their rates" covers this calculation in detail.

6 Chapter summary

Test your understanding answers

Test your understanding 1

Summary of the determinants of option prices.

Comments:

• Share price and exercise price – opposite of call option.
• Time and volatility – same argument as for call.
• Interest rate – a higher interest rate reduces the present value of deferred receipts making the option less valuable as an alternative to selling now.

Test your understanding 2

Buying put options would allow the manager to limit the downside exposure.

Test your understanding 3

Value of a call option = P_a N(d₁) – P_eN(d₂)e^–rt

Step 1: Compute d₁ and d₂.

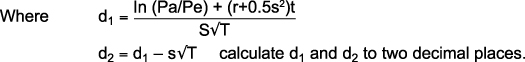
d₁= [ln(164/148) + (0.05 + 0.3464 ² )0.5] / 0.3464 × √0.5 = 0.64

d₂ = 0.64 - (0.3464 × √0.5) = 0.40

Step 2: Compute N(d₁) and N(d₂).

N(d₁) = 0.5 + 0.2389 = 0.7389

N(d₂) = 0.5 + 0.1554 = 0.6554

Step 3: Use formula.

Test your understanding 4

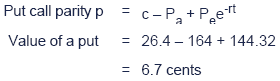
Test your understanding 5

• The present value of the dividends is equal to: 2e^-rt = 2e^{-(0.08 × 0.25)} = 1.96
• So P_a – PV (dividends) = $40.00 – 1.96 = $38.04, is used in the Black-Scholes model.

Test your understanding 6

• Vertical integration is usually evaluated in terms of cost, quality and barriers to entry.
• By outsourcing, the company can switch between different types of supply and different suppliers.
• Vertical integration loses this flexibility, effectively giving up a switching option.

Test your understanding 7

The studio has effectively acquired a learning option allowing bettersubsequent decisions. The feedback generates a range of call options onfuture marketing investment.

Test your understanding 8

The value of the call option on selling gadgets is calculated using Black-Scholes:

Step 1: Compute d₁ and d₂.

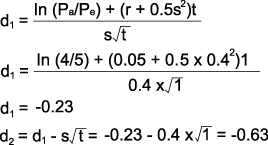
Step 2: Compute N(d₁) and N(d₂).

N(d₁) = 0.5 - 0.0910 = 0.4090

N(d₂) = 0.5 - 0.2357 = 0.2643

Step 3: Use formula.

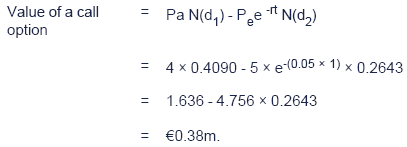
Summary

The project should thus be rejected.

Rating :	Ratings & Comments  (Click the stars to rate the page)

Tags: