The Value of Imperfect Information
A complication that arises with decision trees is that they allow you to calculate the value of having further information, say about market conditions, which in turn allows you to decide whether or not it is worth paying for market research. This page looks at how to value imperfect information
Information
Decision makers may be offered a forecast of a future outcome (for example a market research group may predict the forthcoming demand for a product). This forecast may turn out to be correct or incorrect. This page looks at how to calculate the value of the forecast.
- Perfect information - The forecast of the future outcome is always a correct prediction. If a firm can obtain a 100% accurate prediction they will always be able to undertake the most beneficial course of action for that prediction.
- Imperfect information - The forecast is usually correct, but can be incorrect. Imperfect information is not as valuable as perfect information.
The value of information (either perfect or imperfect) may be calculated as follows:
Expected Profit (Outcome) WITH the information LESS Expected Profit (Outcome) WITHOUT the information
Illustration
(a)You have the mineral rights to a piece of land that you believe may have oil underground. There is only a 10% chance that you will strike oil if you drill, but the profit is $200,000.
It costs $10,000 to drill. The alternative is not to drill at all, in which case your profit is zero.
Should you drill? Draw a decision tree to represent your problem.
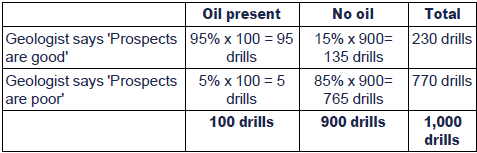
(b) Before you drill, you may consult a geologist who can assess the promise of the piece of land. She can tell you whether the prospects are good or poor, but she is not a perfect predictor. If there is oil, the probability that she will say there are good prospects is 95%. If there is no oil, the probability that she will say prospects are poor is 85%.
Draw a decision tree and calculate the value of imperfect information for this geologist. If the geologist charges $7,000, would you use her services?
Solution
(a)
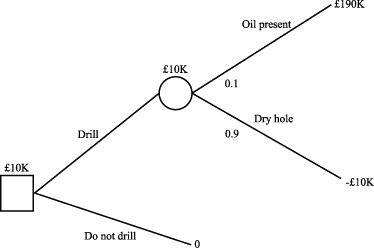
EV ('Drill') = ($190K × 0.1) + (-$10K × 0.9) = $10K.
We should drill, because the expected value from drilling is $10K, versus nothing for not drilling.
(b) We will calculate the Expected Value of profits if we employ the geologist.
If this exceeds $10,000, the geologist would be worth employing as long as the benefit of employing her exceeds her charge of $7,000.
If we employ the geologist, the probabilities of her possible assessments can be tabulated as follows (assume 1,000 drills in total):
A decision tree can be drawn to calculate the expected value of profits if a geologist is employed:
Working from right to left:
EVA = (41.30% × $200,000) - $10,000 drilling costs = $72,600.The decision at 'C' should be to drill, as this generates higher benefits than not drilling.
EVB = (0.65% × $200,000) - $10,000 drilling costs = -$8,700. The decision at 'D' should be not to drill.
EVC = 0.23 × $72,600 = $16,698. This is the expected value of profits if a geologist is employed and exceeds the EV of profits if she is not employed.
Expected Value of Imperfect Information = $16,698 - $10,000 =$6,698. Since this is less than the cost of buying the information($7,000), we should not employ the geologist.
|
Created at 7/10/2012 9:21 PM by System Account
(GMT) Greenwich Mean Time : Dublin, Edinburgh, Lisbon, London
|
Last modified at 4/26/2013 3:46 PM by System Account
(GMT) Greenwich Mean Time : Dublin, Edinburgh, Lisbon, London
|
|
|
|
 |
Rating
:
|
 Ratings & Comments
(Click the stars to rate the page) Ratings & Comments
(Click the stars to rate the page)
|
 |
Tags:
|
|
|
|
|